Partitionierte Lösungsansätze in der Strukturdynamik und der Fluid-Struktur-Interaktion

Mok D.P.

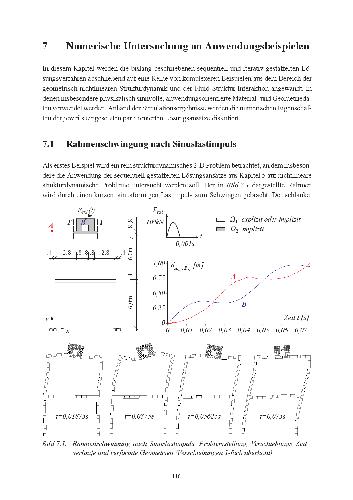
The area of partitioned analysis techniques for the numerical simulation of coupled systems has experienced increasingly intense research efforts in many areas of application over the last few years. This is due to a series of advantages over simultaneous, monolithic solution methods. Partitioned solution approaches allow the independent use of suitable modelling, discretization and solution methods for the individual subsystems, a modular and parallel software architecture, as well as the reduction of the numerical solution and memory efforts by decomposing the fully coupled system into subsystems which are easier to handle separately. The present report studies methods for the partitioned solution of coupled dynamical systems, particularly with regard to geometrically nonlinear structural dynamics and the transient interaction of instationary. incompressible flows with flexible structures featuring large deformations. Formulations developed in different scientific disciplines are compiled within a unified framework and classifying survey. They are differentiated according to the type of spacial partitioning, the time integration methods used in the subsystems, and the coupling strategy, as these criteria are of fundamental relevance for construction and numerical properties of the specific partitioned schemes. Within the class of non-overlapping Dirichlet-Neumann methods, which are especially suitable for the simulation of the problems in mind, simple staggered and iterative staggered coupling strategies, i.e. coupling strategies leading to algorithmically loose and strong coupling, respectively, are further investigated and compared theoretically and numerically. It is shown that simple staggered schemes are numerically cheap and – if formulated appropriately – sufficiently accurate, yet they are in principle weakly unstable. Tins weak instability is caused by their inherent violation of the basic balance equations for mass and energy due to a lack of kinematic continuity at the interface between adjacent subsystems, and amplified by the “artificial added mass”-effect in the case of fluid structure interaction with incompressible flows. Iterations over the subsystems, i.e. iterative staggered schemes, have proven to be the most advisable stabilization technique. These techniques are traced back to classical domain decomposition methods (iterative substructuring methods). By this means it has become possible to derive convergence statements, and to develop efficient, robust and user-friendly convergence acceleration schemes. In particular, the application of the gradient method and Ait-ken’s method are proposed. Finally, a series of numerical examples demonstrates the specific applicability and effectiveness of the analyzed partitioned solution approaches.